Effectiveness
Con ● duc ● tiv ● i ● ty
/ kän dək tivədē/
noun
The measure of a material’s ability to transfer heat.

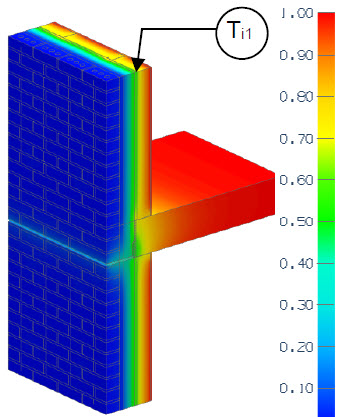
Thermal image of heat loss at concrete balcony connection
Heat Loss
What is the effectiveness of structural thermal breaks at reducing heat loss? The thermal conductivity of a material is an important variable in determining the rate at which heat flows through that material. Heat flow also is dependent on area and temperature. Given the same boundary conditions of, temperature difference across two materials, and the same area and thickness, the material with the higher thermal conductivity will transfer heat at a higher rate.
Thermal breaks are characterized either by their thermal conductivity (k) or thermal resistance (R). The two values are related so either value can be calculated from the other. To determine the effectiveness of a thermal break at reducing heat loss, a thermal model should be created of the detail within the building’s wall or roof assembly. The k or R value of all the materials in the assembly are required in the model.
Why is modeling necessary? Two reasons: First, heat does not flow in parallel paths when highly conductive construction materials are combined in an assembly. If it did, we could use simple math and area-weighted averaging to determine heat flow through an assembly. Second, many interface and transition details are complex and involve corners or other features that make it difficult at best to calculate heat flow.

Consider this:
Steel Z girts can occupy perhaps 10% of a buildings’ exterior wall surface. Yet, they can reduce the clear field R value of a wall by as much as 50%.
Balconies on a building can occupy 3% of the exterior wall surface. It has been shown that balconies can be responsible for as much as 30% of the heat loss in a wall assembly.
Area weighting and parallel heat path assumptions will lead you down an inaccurate path…..
Thermal model of a typical masonry facade assembly using a shelf angle at the floor slab. Note the temperature differences at the angle and within the floor slab.
The results of a thermal model will tell you:
• The actual R value and U value of the assembly without a thermal break
• The adjusted or effective R and U values with a thermal break applied
• How much heat loss is due to the thermal bridging detail
• How much the thermal break improves that heat loss
• The surface temperatures of the materials in the assembly which will indicate whether condensation is likely
To be effective, a thermal break has to have a much, much lower thermal conductivity than the material it is “breaking.” Does thickness matter? In short, yes. For all materials, conductance is a function of thickness. Recall, that heat flow is a function of area, temperature and thermal conductivity. Since the conductance of a material is a function of its thickness, both thickness and area are important in heat flow calculations for a thermal break.
In some instances, using a thermal break that is too thin, can have adverse results! Let’s look at a steel beam supporting a balcony. If we “break” the beam where it passes through the thermal envelope to incorporate a thermal break, we probably need to add an end plate on either side of the break in the beam to connect the thermal break. When we do this, we increase the contact surface area of the steel. Without a thermal break, we would be making the heat flow through the beam worse. With a thermal break, we need to acknowledge that the conductance of the thermal break material is a function of its thickness and the heat flow through the now thermally broken connection is a function of that, plus the area of the connection. Too thin and the impact of the thermal break is lost due to the increase in area of the highly conductive steel. Modeling of several thermal break solutions has shown that the thickness should be at least 1” to achieve any significant reduction in heat loss. This of course does vary by application and assembly.
In any connection design using a thermal break, the goal is to find the appropriate thickness/area combination that helps the wall or roof assembly meet the U value requirement based on climate zone and energy code.
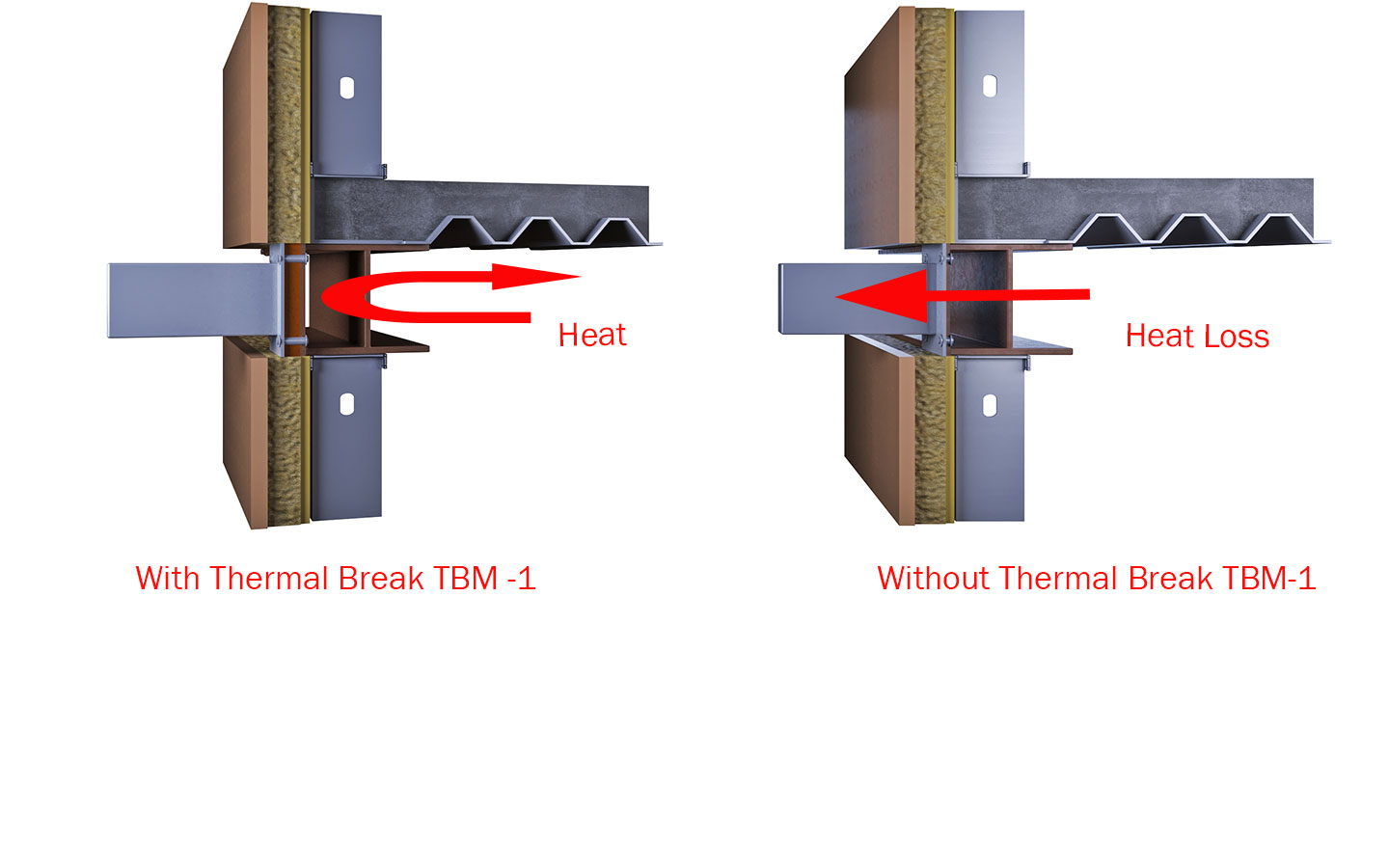
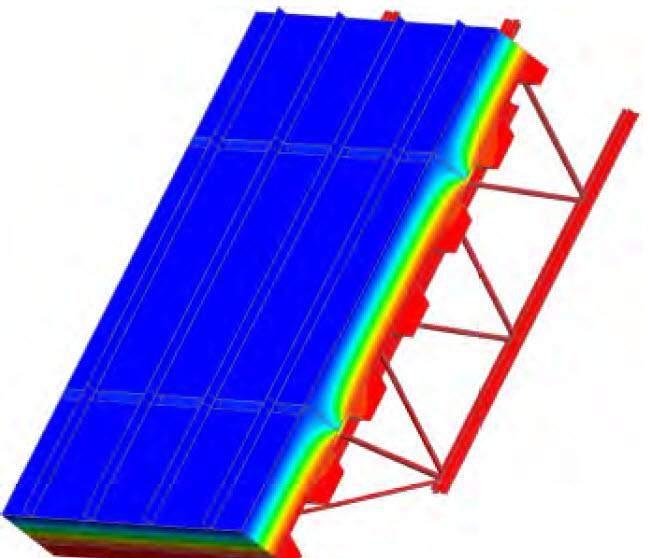
Image Credit: Morrison Hershfield BETBG, Appendix B, Catalogue Material Data Sheets (Version 1.3)
Heat flow associated with steel purlins in a metal building roof. Note the cooler temperatures of the purlin interior surfaces due to thermal bridging.
Condensation
While a properly designed thermal break will considerably reduce heat flow, thermal breaks are also effective at keeping connection surfaces above the dew point. This is particularly important for connection details in buildings where higher than normal relative humidity values exist (hospitals or natatoriums) or in the Southern part of the United States where humidity levels are higher.
A secondary benefit of using a thermal break is to control material surface temperatures. Thermal bridging allows heat from the interior conditioned space to flow out toward the exterior of the enclosure. When the temperature difference is large, interior material surfaces cool. To prevent the potential of condensation forming in the thermal envelope, the surface temperatures of the materials within the envelope must be kept above the dew point temperature. A risk of condensation is possible if thermal bridges that pierce the envelope are not addressed by using a thermal break.